Assignments
computer-controlled cutting (Feb 6)
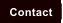
Regular Polyhedra. Platonic Solids Within the laser cutting exercise I perform a practice to bring 3D geometry to schoolchildren in Seville.
Geometry, spatial vision, the concept of the three-dimensional display is not welcomed
schoolchildren in Seville.
Geometry, spatial vision, the concept of the three-dimensional display is not welcomed because of new technologies (film, television, video games). Educational programs have
because of new technologies (film, television, video games). Educational programs have not assimilated this concept until an advanced age and curricula already directed
not assimilated this concept until an advanced age and curricula already directed towards technical studies.
The purpose of this practice, focused on the development of Computer-Controlled
towards technical studies.
The purpose of this practice, focused on the development of Computer-Controlled Cutting and regarding my final work is focused on the development of the five regular
Cutting and regarding my final work is focused on the development of the five regular polyhedra exist: hexahedron, icosahedron, dodecahedron, octahedron and tetrahedron.
polyhedra exist: hexahedron, icosahedron, dodecahedron, octahedron and tetrahedron.
 This practice is aimed at children aged 10-14 years, an age where no special requires
This practice is aimed at children aged 10-14 years, an age where no special requires geometric knowledge in schools, but the kids interested and want to start learning more.
In design practice, a historical approach allows children to know that regular polyhedra
geometric knowledge in schools, but the kids interested and want to start learning more.
In design practice, a historical approach allows children to know that regular polyhedra were known in ancient Greece. Com that Plato used symbols of his philosophy, and
were known in ancient Greece. Com that Plato used symbols of his philosophy, and each represents earth, air, fire and water, combining the tetrahedron to the fifth element
each represents earth, air, fire and water, combining the tetrahedron to the fifth element or spiritual entity.
or spiritual entity. I get to practice with children who talk philosophy and mathematics, but without being
I get to practice with children who talk philosophy and mathematics, but without being heavy for them.
heavy for them.
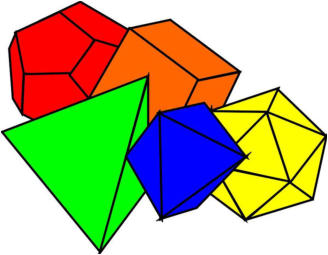
 The amount of software to represent geometric figures is wide. In Andalusia schools
The amount of software to represent geometric figures is wide. In Andalusia schools students have computers running Ubuntu which include this software. But for the
students have computers running Ubuntu which include this software. But for the development of this practice is better than software suits Fab Academy.
development of this practice is better than software suits Fab Academy.
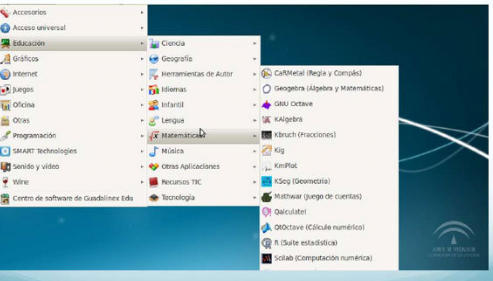
 In the example shown in the image, use the golden rectangle to generate an
In the example shown in the image, use the golden rectangle to generate an icosahedron from Rhinoceros. It is a quick exercise to show students.
icosahedron from Rhinoceros. It is a quick exercise to show students.
 The development of laser cutting exercise I have chosen the program Inkscape, which
The development of laser cutting exercise I have chosen the program Inkscape, which helps with your clone tool to play with the tabs and get a correct fit of the faces of the
helps with your clone tool to play with the tabs and get a correct fit of the faces of the polyhedra.
polyhedra.
 In developing the model, the part that I'm most happy is the union of the edges,
In developing the model, the part that I'm most happy is the union of the edges, developed to serve in the construction of all regular polyhedra, preventing exists a model
developed to serve in the construction of all regular polyhedra, preventing exists a model for every angle, all being in the same room , saving material and space for a more
for every angle, all being in the same room , saving material and space for a more elaborate design generate a wrapper that allows to keep all the pieces together to store
elaborate design generate a wrapper that allows to keep all the pieces together to store or move.
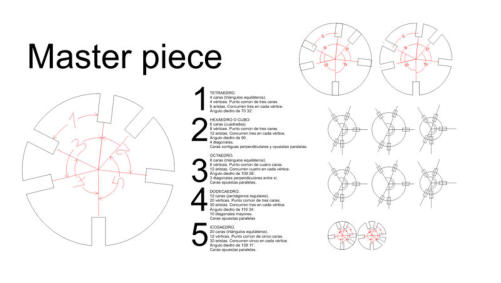
or move. The circular piece is marked in each angle corresponding to each polyhedron and
The circular piece is marked in each angle corresponding to each polyhedron and facilitates assembly of the final model using the following numerical relationship:
facilitates assembly of the final model using the following numerical relationship:
 Piece nº 1. Tetrahedron.
Piece nº 1. Tetrahedron. 4 faces (equilateral triangles).
4 vertices. Common point of three faces.
4 faces (equilateral triangles).
4 vertices. Common point of three faces. 6 edges. Concurren three at each vertex.
6 edges. Concurren three at each vertex. Dihedral angle of 70 32 '.
Dihedral angle of 70 32 '. Piece nº 2. Cube.
Piece nº 2. Cube. 6 guys (cuadrados).
8 vertices. Common point of three faces.
6 guys (cuadrados).
8 vertices. Common point of three faces. 12 edges. Concurren three at each vertex.
12 edges. Concurren three at each vertex. Dihedral angle of 90.
Piece nº 3. Octahedron.
Dihedral angle of 90.
Piece nº 3. Octahedron. 8 faces (equilateral triangles).
6 vertices. Common point of four faces.
8 faces (equilateral triangles).
6 vertices. Common point of four faces. 12 edges. Concurren four at each vertex.
12 edges. Concurren four at each vertex. Dihedral angle of 109 28 '.
Dihedral angle of 109 28 '. Piece nº 4. Dodecahedron.
Piece nº 4. Dodecahedron. 12 faces (regular pentagons).
20 vertices. Common point of three faces.
12 faces (regular pentagons).
20 vertices. Common point of three faces. 30 edges. Concurren three at each vertex.
30 edges. Concurren three at each vertex. Dihedral angle of 116 34 '.
Piece nº 5. Icosahedron.
Dihedral angle of 116 34 '.
Piece nº 5. Icosahedron. 20 faces (equilateral triangles).
12 vertices. Common point of five guys.
20 faces (equilateral triangles).
12 vertices. Common point of five guys. 30 edges. Concurren five in each vertex.
30 edges. Concurren five in each vertex. Dihedral angle of 138 11 '.
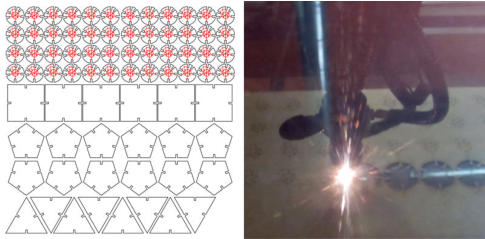
Modelo preparado para corte:
Dihedral angle of 138 11 '.
Modelo preparado para corte:
 During design development, the main problem I had was choosing the sizes of the tabs
During design development, the main problem I had was choosing the sizes of the tabs and their number per edge. I chose 3 mm MDF material to cut and started designing
and their number per edge. I chose 3 mm MDF material to cut and started designing parts with two tabs per edge. These two faces are oversized for the final result, so
parts with two tabs per edge. These two faces are oversized for the final result, so eyelashes went from two to one per edge.
eyelashes went from two to one per edge.
 The result is a pattern of regular polyhedra physical representation that allows the child
The result is a pattern of regular polyhedra physical representation that allows the child to play with their mounting, known characteristics of each (sides, edges, angles, corners,
to play with their mounting, known characteristics of each (sides, edges, angles, corners, etc.) and be the beginning of the spatial geometry designed by computer.
etc.) and be the beginning of the spatial geometry designed by computer.  Algunos ejemplos de las piezas terminadas:
Algunos ejemplos de las piezas terminadas:


 To improve the design:
- Container box and engraved on the faces of the polyhedra characteristics and number.
To improve the design:
- Container box and engraved on the faces of the polyhedra characteristics and number. - Increased size of the faces.
- Increased size of the faces. Software used:
- Photoshop.
- Inkscape
- Gimp
- Rhinoceros
- sketchup
Software used:
- Photoshop.
- Inkscape
- Gimp
- Rhinoceros
- sketchup







Juan Carlos Pérez Juidias. Fab Lab Escuela Técnica Superior de Arquitectura de la Universidad de Sevilla